Trigonometri Kelas 10

Berikut ringkasan materi trigonometri kelas 10 :

Radians - an Alternative Measure for Angle

In science and engineering, radians are much more convenient (and common) than degrees. A radian is defined as the angle between 2 radii (radiuses) of a circle where the arc between them has length of one radius.
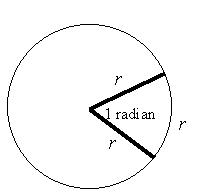
Another way of putting it is: "a radian is the angle subtended by an arc of length r (the radius)".
One radian is about 57.
Radians are especially useful in calculus where we want to interchange angles and other quantities (e.g. length). For example, see how radians are required in Fourier Series. That stuff won't work if we try to use degrees.

Most computer programs use radians as the default.
Care with your calculator! Make sure your calculator is set to radians when you are making radian calculations.
Also, see this simple introduction to radians with an interactive graph.

Converting Degrees to Radians

Because the circumference of a circle is given by C = 2πr and one revolution of a circle is 360°, it follows that:

2π radians = 360°.

This gives us the important result:

π radians = 180°

From this we can convert:

radians → degrees and

degrees → radians.

PENJUMLAHAN DUA SUDUT (a + b)

sin(a + b)  = sin a cos b + cos a sin b
cos(a + b) = cos a cos b - sin a sin b
tg(a + b )   = tg a + tg b
                 1 - tg²a

SELISIH DUA SUDUT (a - b)

sin(a - b)  = sin a cos b - cos a sin b
cos(a - b) = cos a cos b + sin a sin b
tg(a - b )   = tg a - tg b
                 1 + tg²a

SUDUT RANGKAP

sin 2a  = 2 sin a cos a
cos 2a = cos²a - sin² a
= 2 cos²a - 1
= 1 - 2 sin²a
tg 2a  =  2 tg 2a 
            1 - tg²a
sin a cos a = ½ sin 2a
cos²a = ½(1 + cos 2a)
sin2a  = ½ (1 - cos 2a)

Secara umum :

sin na  = 2 sin ½na cos ½na
cos na = cos² ½na - 1
= 2 cos² ½na - 1
= 1 - 2 sin² ½na
tg na =   2 tg ½na  
           1 - tg² ½na

JUMLAH SELISIH DUA FUNGSI YANG SENAMA


BENTUK PENJUMLAHAN ® PERKALIAN

sin a + sin b   = 2 sin a + b    cos a - b
                                2              2
sin a - sin b   = 2 cos a + b    sin a - b
                                2             2
cos a + cos b = 2 cos a + b    cos a - b
                                 2              2
cos a + cos b = - 2 sin a + b   sin a - b
                                  2             2

BENTUK PERKALIAN ® PENJUMLAHAN

2 sin a cos b = sin (a + b) + sin (a - b)
2 cos a sin b = sin (a + b) - sin (a - b)
2 cos a cos b = cos (a + b) + cos (a - b)
- 2 sin a cos b = cos (a + b) - sin (a - b)

PENJUMLAHAN FUNGSI YANG BERBEDA

Bentuk a cos x + b sin x

Merubah bentuk a cos x + b sin x ke dalam bentuk K cos (x - a)

a cos x + b sin x = K cos (x-a)

dengan :                     
             K = Öa² + b² dan tg a = b/a Þ a = ... ?

Kuadran dari a ditentukan oleh kombinasi tanda a dan b sebagai berikut

	I	II	III	IV
a	+	-	-	+
b	+	+	-	-

keterangan :
a = koefisien cos x
b = koefisien sin x 

Sumber : http://bebas.ui.ac.id/v12/sponsor/Sponsor-Pendamping/Praweda/Matematika/0430%20Mat%203-1g.htm



http://www.intmath.com/trigonometric-functions/7-radians.php